Exercise 20A
Question 1. The height of a circular cylinder is 20 cm and the radius of its base is 7 cm. Find:
(i) the volume
(ii) the total surface area.
Solution:
Given that, Height of the cylinder (h) = 20cm
Radius of the base of the cylinder (r) = 7cm

Question 2. The inner radius of a pipe is 2.1 cm. How much water can 12m of this pipe hold?
Solution:
It is given that, radius of pipe = 2.1cm and length of the pipe = 12m = 1200cm.
Volume of the cylinder = πr2 h
Volume of the cylinder = 22/7× (2.1)2 1200
Volume of the cylinder = 16632cm3
Hence, the pipe can hold 16632cm3 of water.
Question 3. A cylinder of circumference 8cm and length 21cm rolls without sliding for 4(1/2) second at the rate of 9 complete rounds per second. Find:
(i) distance travelled by the cylinder in 4(1/2) seconds and
(ii) the area covered by the cylinder in 4(1/2) seconds.
Solution:
(i) It is given that, the circumference of cylinder = 8cm, then distance covered in 9 revolutions = 9 × 8 = 72cm.
Distance covered in 1 second = 72cm

Question 4. How many cubic meters of earth must be dug out to make a well 28m deep and 2.8m in diameter? Also, find the cost of plastering its inner surface at Rs. 4.50 per sq meter.
Solution:
It is given that, the diameter of the wall is 2.8m
Radius of the wall = 2.8/2
Radius of the wall = 1.4m
Depth of the wall = 28m
Volume of earth dug out = πr2 h

Question 5. What length of solid cylinder 2 cm in diameter must be taken to recast into a hollow cylinder of external diameter 20 cm, 0.25 cm thick and 15 cm long?
Solution:
It is given that, the external diameter of hollow cylinder = 20cm
Radius = 20/2
Radius = 10cm
Thickness = 0.25cm
Internal radius = 10 – 0.25
Internal radius = 9.75cm
Length of the cylinder (h) = 15cm
Volume of the cylinder = πh(R2 – r2)
Volume of the cylinder = π×15((10)2 – (9.75)2 )
Volume of the cylinder = 15π(100 – 95.0625)
Volume of the cylinder = 15π(4.9375)
Diameter = 2cm
Radius = 1cm
Let us assumed that, h be the length,
Volume = πhr2
Volume = πh(1)2
Volume = πh
According to question,
πh = 15π(4.9375)
h = 15(4.9375)
h = 74.0625cm
Hence, the length of the cylinder is 74.0625cm.
Question 6. A cylinder has a diameter of 20 cm. The area of curved surface is 100 sq. cm. Find:
(i) the height of the cylinder correct to one decimal place.
(ii) the volume of the cylinder correct to one decimal place.
Solution:
(i) It is given that, the diameter of the cylinder = 20cm
Radius = 10cm
Height = hcm
Curve Surface Area = 2πrh
100cm2 = 2πrh
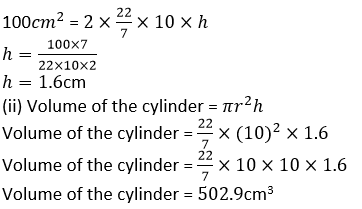
Question 7. A metal pipe has a bore (inner diameter) of 5 cm. The pipe is 5 mm thick all round. Find the weight, in kilogram, of 2 metres of the pipe if 1 cm3 of the metal weights 7.7g.
Solution:
It is given that, Inner radius of the pipe (r) = 5/2
Radius = 2.5 cm
External radius of the pipe (R) = Inner radius of the pipe + Thickness of the pipe
External radius of the pipe (R) = 2.5cm + 0.5cm
External radius of the pipe (R) = 3cm
Length of the pipe (h) = 2m
Length of the pipe = 200cm
Volume of the pipe = External Volume – Internal Volume
Volume of the pipe = πR2 h – πr2 h
Volume of the pipe = πh(R2 – r2 )
Volume of the pipe = π×200×((3)2 – (2.5)2 )
Volume of the pipe = 200π(9 – 6.25)
Volume of the pipe = 200π(2.75)
Volume of the pipe = 200×22/7×(2.75)
Volume of the pipe = 1728.6cm3
1cm3of the metal weights 7.79,
Weight of the pipe = (1728.6 × 7.7)g
Weight of the pipe = (1728.6×7.7/1000)kg
Weight of the pipe = 13.31 kg
Question 8. A cylindrical container with diameter of base 42 cm contains sufficient water to submerge a rectangular solid of iron with dimensions 22cm × 14cm × 10.5cm. Find the rise in level of the water when the solid is submerged.
Solution:
It is given that, diameter of cylindrical container = 42cm
Radius (r) = 21cm
The dimensions of rectangle solid = 22cm × 14cm × 10.5cm
Volume of the solid = 22cm × 14cm × 10.5cm
Volume of water in the container = πr2 h
22/7×(21)2×h = 22cm × 14cm × 10.5cm
22×3×21×h = 22cm×14cm×10.5cm
63h = 14cm×10.5cm
h = 14cm×10.5cm/63
h = 7/3
h = 2.33cm
Question 9. A cylindrical container with internal radius of its base 10cm, contains water up to a height of 7 cm. Find the area of wetted surface of the cylinder.
Solution:
It is given that, the internal radius of the cylindrical container = 10cm
Height of water = 7cm
Surface area of the wetted surface = 2πh+πr2
Surface area of the wetted surface = πr(2h+r)
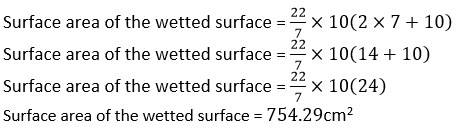
Question 10. Find the total surface area of an open pipe of length 50 cm, external diameter 20 cm and internal diameter 6cm.
Solution:
It is given that, Length of the open pipe = 50cm
External diameter (d) = 20cm
External radius (r) = 20/2 = 10cm
Internal diameter (d) = 6cm
Internal radius (r) = 6/2 = 3cm
Surface area of pipe open from both sides = 2πRh + 2πrh
Surface area of pipe open from both sides = 2πh(R+r)

Question 11. The height and radius of base of cylinder are in the ratio 3:1. If its volume is 1029π cm3, find its total surface area.
Solution:
It is given that, the ratio between height and radius of a cylinder = 3:1
Volume = 1029π cm3________(i)
Let us assumed that, radius of the base = r
Height = 3r
Volume of the cylinder = πr2 h
Volume of the cylinder = π×r2×3r
Volume of the cylinder = 3πr3_______(ii)
From equation (i) and (ii)
3πr3 = 1029π
r3 = 1029/3
r3 = 343
r = 3√343
r = 7cm
Height of the cylinder = 3×7
Height of the cylinder = 21cm
Total surface area = 2πr(h+r)
Total surface area = 2×22/7×7(21+7)
Total surface area = 2×22×(28)
Total surface area = 1232cm2
Question 12. Find the minimum length in cm and correct to nearest whole number of the thin metal sheet required to make a hollow and closed cylindrical box of diameter 20cm and height 35cm. Given that the width of the metal sheet is 1 m. Also, find the cost of the sheet at the rate of Rs. 56 per m.
Find the area of metal sheet required, if 10% of it is wasted in cutting, overlapping, etc.
Solution:
It is given that, the height of the cylindrical box (h) = 35cm
Radius of the cylindrical box (r) = 10cm
Width of the sheet = 1m = 100cm
Area of sheet = Total surface area of the box
Length × Width = 2πr(r+h)
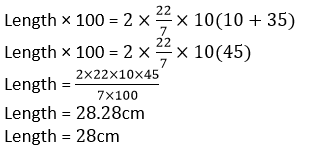
Area of the metal sheet = Length × Width
Area of the metal sheet = 28 × 100
Area of the metal sheet = 2800cm2
Area of the metal sheet = 00.28m2
It is given that, cost of the sheet at the rate of Rs. 56 per m2
Cost of the sheet = Rs. 56 × 0.28
Cost of the sheet = Rs. 15.68

Question 13. 3080cm3 of water is required to fill a cylindrical vessel completely and 2310 cm3 of water is required to fill it upto 5 cm below the top. Find:
(i) radius of the vessel.
(ii) height of the vessel.
(iii) wetted surface area of the vessel when it is half-filled with water.
Solution:
Let us assumed that, the radius of the cylindrical vessel be r and height be h.
Volume of cylindrical vessel = Volume of water filled
πr2 h=3080

Question 14. A metal cube of side 11 cm is completely submerged in water contained in a cylindrical vessel with diameter 28 cm. Find the rise in the level of water.
Solution:
Here,
Volume of the cube = Volume of water displaced in the cylinder
(11)3 = πr2 h
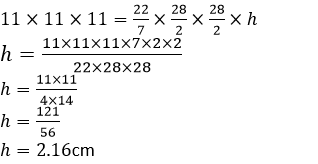
Question 15. A circular tank of diameter 2m is dug and the earth removed is spread uniformly all around the tank to form an embankment 2m in width and 1.6m in height. Find the depth of the circular tank.
Solution:
Let us assumed that, the depth of the circular tan be hm.
Diameter of the tank = 2m
Radius of the tank = 2/2 = 1m
Volume of the tank = πr2 h
Volume of the tank = π×(1)2 h
Volume of the tank = πh m3
Volume of the embankment = Volume of hollow cylinder having height 1.6m
Volume of the embankment = πh(R2 – r2)
Volume of the embankment = π(1.6)(32 – 12)
Volume of the embankment = π(1.6)(9 – 1)
Volume of the embankment = π(1.6)(8)
Volume of the embankment = 12.8πm3
Volume of tank = Volume of embankment
πh = 12.8π
h = 12.8m
Question 16. The sum of the heights and the radius of a solid cylinder is 35 cm and its total surface area is 3080 cm2, find the volume of the cylinder.
Solution:
Let us assumed that, r is radius and h be the height of a solid cylinder.
r + h = 35cm
Total surface area of a cylinder = 3080cm2
2πr(h + r) = 3080
2×22/7×r(35) = 3080
2×22×r(5) = 3080
r = 3080/(2×22×5)
r = 14cm
It is given that,
14 + h = 35cm
h = 35-14
h = 21cm
Volume of cylinder = πr2 h
Volume of cylinder = 22/7×(14)2×21
Volume of cylinder = 22/7×14×14×21
Volume of cylinder = 22×2×14×21
Volume of cylinder = 12936cm2
Question 17. The given figure shows a solid formed of a solid cube of side 40cm and a solid cylinder of radius 20cm and height 50cm attached to the cube as shown. Find the volume and the total surface area of the whole solid (Take π = 3.14)

Solution:
It is given that, Edge of a cube (l) = 40cm
Volume of a cube l3= (40)3
Volume of a cube l3= 64000cm3
Radius of a solid cylinder (r) = 20cm
Height of a solid cylinder (h) = 50cm
Volume of cylinder = πr2 h
Volume of cylinder = 22/7×(20)2×50
Volume of cylinder = 22/7×20×20×50
Volume of cylinder = 62800cm3
Volume of the solid = Volume of cube + Volume of cylinder
Volume of the solid = 64000 + 62800
Volume of the solid = 126800cm3
Total surface area of the whole solid = Total surface area of a cube + Curve surface area of cylinder
Total surface area of the whole solid = 6l2 + 2πrh
Total surface area of the whole solid
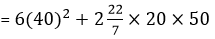
Total surface area of the whole solid = 6(1600) + 6280
Total surface area of the whole solid = 9600 + 6280
Total surface area of the whole solid = 15880cm2
Question 18. A dosed cylindrical tank, made of thin iron sheet, has diameter = 8.4m and height 5.4m. How much metal sheet, to the nearest m2, is used in making this tank, if 1/15 of the sheet actually used was wasted in making the tank?
Solution:
It is given that, the diameter of the cylindrical tank = 8.4/2 = 4.2m
Height of the cylindrical tank (h) = 5.4m
Total surface area of the cylindrical tank = 2πr(h + r)
Total surface area of the cylindrical tank = 2×22/7×4.2(5.4 + 4.2)
Total surface area of the cylindrical tank = 2×22×0.6(9.6)
Total surface area of the cylindrical tank = 253.44m2
Area of sheet wasted in making the tank = 1/15×253.44
Area of sheet wasted in making the tank = 16.896m2
Total sheet required = 253.44 + 16.896 = 270.34m2
Exercise 20 B
Question 1. Find the volume of a cone whose slant height is 17 cm and radius of base is 8 cm.
Solution:
It is given that, Slant height (l) = 17cm
Radius (r) = 8cm
l2 = r2 + h2
(17)2 = (8)2 + h2
289 = 64 + h2
h2 = 289-64
h2 = 225
h = √225
h = 15
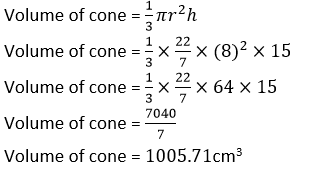
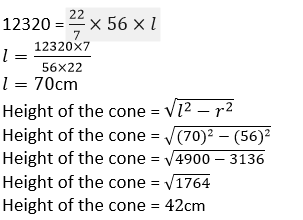
Question 2. The curved surface area of a cone is 12320cm2. If the radius of its base is 56cm, find its height.
Solution:
It is given that, the Curve surface area = 12320cm2 and the radius of base (r) = 56cm
Let us assumed that, the slant height = l
Curve surface area = πrl
12320 = πrl
Question 3. The circumference of the base of a 12 m high conical tent is 66 m. Find the volume of the air contained in it.
Solution:
It is given that the, Circumference of conical tent = 66cm
Height (h) = 12m
Circumference of circle = 2πr
2πr = 66

Question 4. The radius and height of a right circular cone are in the ratio 5:12 and its volume is 2512 cubic cm. Find the radius and slant height of the cone. (Take π = 3.14)
Solution:
It is given that, the ratio between radius and height = 5:12 and Volume = 2512 cubic cm
Let us assumed that, radius (r) = 5x and height (h) = 12x and slant height = l
l2 = r2 + h2
l2 = (5x)2 + (12x)2
l2 = 25x2 + 144x2
l2 = 169x2
l = √169x2
l = 13x
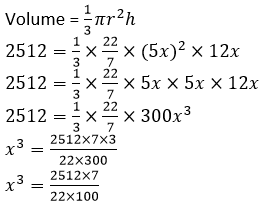
x3 = 8
x = 2
Radius = 5x
Radius = 5×2
Radius = 10cm Height = 12x
Height = 12×2
Height = 24cm Slant Height = 13x
Slant Height = 13×2
Slant Height = 26cm
Question 5. Two right circular cones x and y are made, x having three times the radius of y and y having half the volume of x. Calculate the ratio between the heights of x and y.
Solution:
Let us assumed that, radius of cone y be r so, radius of cone x = 3r
Also, volume of cone y be v so, radius of cone x = 2v
Here, Height of the cone x = h1 and Height of the cone y = h2

Question 6. The diameters of two cones are equal. If their slant heights are in the ratio 5:4, find the ratio of their curved surface areas.
Solution:
It is given that, the ratio of slant height = 5:4.
Let us assumed that, radius of each cone = r, Height of first cone = 5x and Height of second cone = 4x
Curved surface area = πrl
Curved surface area of first cone = πr(5x)
Curved surface area of first cone = 5πrx
Curved surface area of second cone = πr(4x)
Curved surface area of second cone = 4πrx
Ratio between Curved surface area = 5πrx/4πrx
Ratio between Curved surface area = 5:4.
Question 7. There are two cones. The curved surface area of one is twice that of the other. The slant height of the latter is twice that of the former. Find the ratio of their radii.
Solution:
Let us assumed that, the height of the first cone = l
Slant height of the second cone = 2l
Radius of the first cone = r1
Radius of the first cone = r2
Curve surface area of first cone = πr1 l
Curve surface area of first cone = πr2 (2l) = 2πr2 l
According to question,
Question 8. A heap of wheat is in the form of a cone of diameter 16.8 m and height 3.5 m. Find its volume. How much cloth is required to just cover the heap?
Solution:
It is given that, the diameter of the cone = 16.8m and radius the circle (r) = 16.8/2 = 8.4m
Height (h) = 3.5m
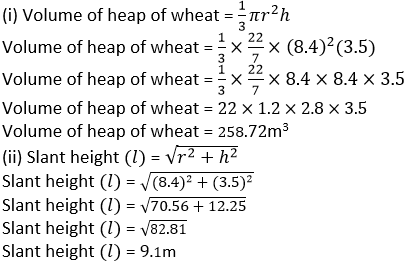
Cloth required or curved surface area = πrl
Cloth required or curved surface area = 22/7×8.4×9.1
Cloth required or curved surface area = 22×1.2×9.1
Cloth required or curved surface area = 240.24m2
Question 9. Find what length of canvas, 1.5m in width, is required to make a conical tent 48m in diameter and 7m in height. Given that 10% of the canvas is used in folds and stitching. Also, find the cost of the canvas at the rate of Rs. 24 per meter.
Solution:
It is given that, Diameter of the tent = 48m
Radius (r) = 24m and Height (h) = 7m
Here,
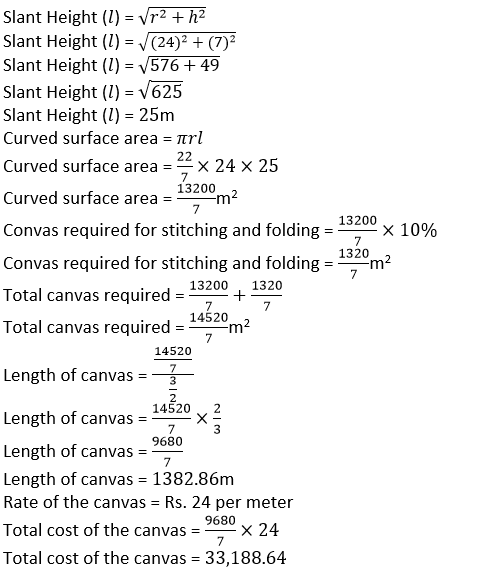
Question 10. A solid cone of height 8cm and base radius 6cm is melted and re-casted into identical cones, each of height 2cm and diameter 1cm. Find the number of cones formed.
Solution:
It is given that, the height of the cone (h) = 8cm
Radius (r) = 6cm
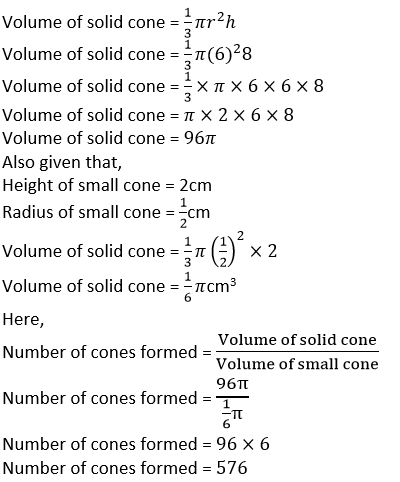
Question 11. The total surface area of a right circular cone of slant height 13 cm is 90π cm2. Calculate:
(i) its radius in cm
(ii) its volume in cm3 take (π = 3.14)
Solution:
(i) It is given that, total surface area of cone = 90π cm2 and slant height (l) = 13cm
Let us assumed that the radius be r.
Total surface area = πrl + πr2
Total surface area = πr(l + r)
90π = πr(l + r)
90 = r(13 + r)
r2 + 13r – 90 = 0
r2 + 18r – 5r – 90 = 0
r(r + 18) – 5(r + 18) = 0
(r – 5)(r + 18) = 0 r – 5 = 0
r = 5 r + 18 = 0
r = – 18
Radius can’t be negative.
Hence, the radius is 5
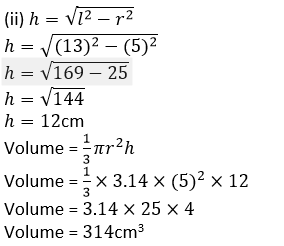
Question 12. The area of the base of a conical solid is 38.5cm2 and its volume is 154cm3. Find the curved surface area of the solid.
Solution:
It is given that, the area of the base (πr2) = 38.5cm2 and the volume is 154cm3.
We know that,
πr2 = 38.5
3.14×r2 = 38.5
r2 = 38.5/3.14
r2 = 12.26
r = √12.26
r = 3.5
Volume of the cone = 1/3 πr2 hcm3
154×3 = 38.5×h
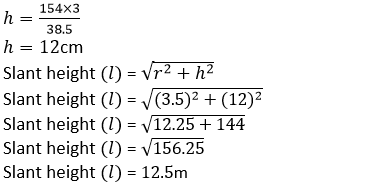
Curved surface area of the solid = πrl
Curved surface area of the solid = 3.14×3.5×12.5
Curved surface area of the solid = 137.44cm2



