Students of ICSE Class 10 should refer to Previous Year Questions ICSE Class 10 Mathematics Banking below which have come in past board exams. You should always go through questions that have come in previous years. This will help you to understand the pattern of questions in ICSE Class 10 History and prepare accordingly. This will help you to get better marks in ICSE Class 10 Board Exams
Previous Year Questions ICSE Class 10 Mathematics Banking
Short Answer Type Questions I
Question: Mr. Jacob has a two years recurring deposit
account in State Bank of India and deposits 1500 per month. If he receives 37,875 at the time of
maturity, find the rate of interest.
Answer: P = 1,500, M.V. = 37,875, n = 24, R = ?
M.V. = P n + P n ( n + 1) × R / 2400
37875 = 1 500 X 24 +
1500 X 24 X 5 X R / 2400
37875 = 36000 + 375R
375R = 37875 – 36000
375R = 1875
R = 1875 / 375
R = 5%
Question: Mohan has a recurring deposit account in a bank for 2 years at 6% p.a. simple interest. If he gets
₹ 1200 as interest at the time of maturity, find :
(i) the monthly instalment
(ii) the amount of maturity
Answer:
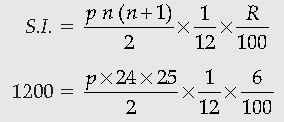
Monthly instalment p = ₹ 800
Maturity value = p × n + S.I. = 800 × 24 + 1200
= ₹ 20400
684 = 342r / 2400 X 800
r = 684 X 2400 / 342 X 800
r = 6%
Question: Shahrukh opened a recurring deposit account in a bank and deposit 8,00 per month for 1 (1 / 2) years. If he received15,084 at the time of maturity, find the rate of interest per annum.
Answer: p = ` 8,00, n = 18, M.V. = ` 15,084
I = pn(n + 1) X 1 / 12 X R / 100
= 800 X 18 X19 / 2 X 1 / 12 X R / 100
= 114 R
Maturity value = p × n + Interest
15084 = 800 × 18 + 114 R
R = 15084 – 14400 / 114 = 684 / 114
= 6%
Detailed Answer : P = ` 8,00
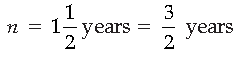
= 18 Months
Maturity value = ₹ 15084
Maturity value = P X n + P X n (n + 1) X r 2400
15,084 = 800 X 18 + 800 X 18 (19) X r /2400
15,084 = 14 400 + 342r X 800 / 2400
15084 – 14400 = 342r X 800 / 2400
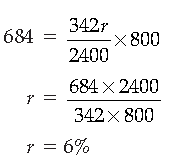
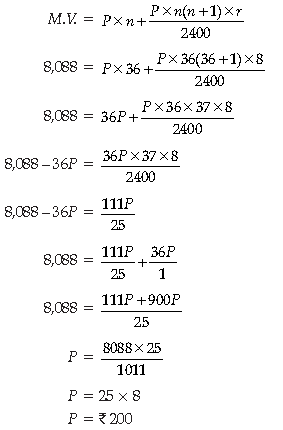
Question: Mr. Britto deposits a certain sum of money each month in a recurring deposit account of a bank.
If the rate of interest is of 8% per annum and Mr. Britto gets ₹ 8,088 from the bank after 3 years, find the value of his monthly instalment.
Answer: R = 8%
M.V. = ₹ 8,088
n = 3 years
= 36 months
Question: Kiran deposited ` 200 for 36 months in a bank recurring deposit account. If the bank pays interest
at the rate of 11% per annum, find the amount she gets on maturity.
Answer: P = ` 2,00
n = 36 months
R = 11%
M.V. = ?
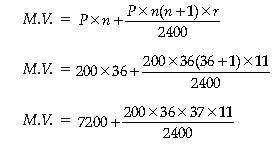
M.V. = 7200 + 33 × 37
M.V. = 7200 + 1221
M.V. = ` 8,421
Question; Ahmad has a recurring deposit account in a bank.
He deposit ₹ 2,500 per month for 2 years. If he gets ₹ 66,250 at the time of maturity, find
(i) the interest paid by the bank
(ii) the rate of interest
Answer: P = ₹ 2500
n = 24 months
M.V. = ₹ 66250
(i) Money deposited = p × n
= 2500 × 24
= 60,000
= Maturity value
– Money deposited
= 66250 – 60000
= ₹ 6,250
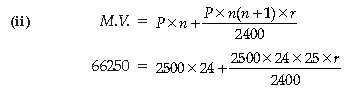
66250 = 60000 + 625r
66250 – 60000 = 625r
6250 = 625r
6250 / 625 = r
r = 10%



